Acronym Finder

In theory, the complexity of computing NP is one of the most fundamental classes. NP stands for “non deterministic polynomial”, which is the current implementation it refers.
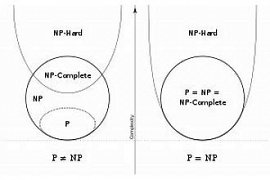
NP set all issues, decisions that find the answer, yes, for they include the simple proof that the answer really should be yes. Generally, the more accurate the proof simple Should to be investigated at a time, run a few sentences in a Turing machine algebras. In contrast to this definition, NP set, issues a decision, which is called a run time polynomial in a Turing machine, non-algebraic evaluation. Class complexity P one of the members of NP, but NP contains the class, another important as well. That is the most complex, they are NP-Complete is as to them no algorithm known runs in time polynomial does not exist .
the most important question that now, for the classes in this theory is whether P=NP a? This question asks whether such an algorithm really matters, NP-Complete and total NP there or not. This widespread belief out there that this equality can not be true....

Catalytic cracking fluidized bed is one of the most important units and conversion processes catalytic in the world is considered beyond heavy materials and low-value oil to the lighter and more valuable conversion. Nowadays, due to increased fuel consumption in the world and need to convert the heavy material to fuel the style need to this process over pre-felt....

Phrase that in the project are very dealt with it. The list of MTO is a list of material required for the construction of a furnishing or structure, etc., where the list of material along with the amount needed and free it (like a certain type of steel, etc.) mentioned. Sometimes this list MTOL also say.
this list is only limited to the amount of material is not required, and the weight of the items that must be shipped or removed (Take Off) is also mentioned in it. This information is an important factor when working with the material, Heavy, is, because to the company carry the material, this will allow the total weight of the items in the list to the fullest, and based on that about how to carry it after making the decision....

Principles of Arc Welding, tungsten covered gas saver
In this process, the practice of welding by the heat arising from electric arc between an electrode consumption of pleasure of tungsten (or alloy) and the workpiece are done. Electrodes and ... electric arc, and area, a pond, molten by a shielding gas (argon and. helium. mix both of the gas or mixture of each of the two gas with hydrogen gas) against the atmosphere to be protected. The use of the gases argon and helium due to the property of being neutral the gases.Gases, neutral with other elements ability to react are not, so in order to remove gases active, such as oxygen and nitrogen, from around the arches and ponds melt, etc. oxides and metal (Porosity), the ideal can be so can be seen from the figure, taking the observed porosities gas stop. The observed porosities, gas, etc. oxides and metal defects are, which reduces the mechanical properties of welding, including resistance to impact and tensile strength....

Eco-friendly (organic compounds of tin with the name of Tri-butyl tin (TBT)) a chemical substance or microorganism which can have any harmful organism by use of a method, chemical or biological means. neutral or under the influence of the controller. Eco-friendly cache, usually in medicine, agriculture, forestry and industry application....

Service distribution data...

A system of Telecommunication for the public data can be used and after doing some processing, and convert the frequency data and sends the same action for Inverse in the receiver....

Surveyor around Mars (MGS) from 1997 up to now, over the Mars and so far more than 20 thousand times round the planet rotated. Make sure all this turnover time is more than 120 thousand image from different regions of Mars. Cameras strong the surveyor pictures over very detailed, from the surface of Mars, which have that resolution, some of them the size of the East that can be a complication to the size of a minibus! On it diagnosed. MGS won the first Atlantic across Mars to prepare.

although the orbiter from all the planet the photo is taken, but photos attention that resolution high have and read more, it turns out. This type of pictures of pretty places of Mars taken, but not from all of the places that is necessary.

to make optimal use of it, ready and keen on Mars, managers plan to think about the acceptance of the order and offer photos of the people fell. Namely, Can anyone to tell them that to a certain reason, and because a certain probability of a certain region, and MGS photos. All the alternatives to shooting, and the attributes are checked and if accepted., at any time, that the orbiter from the top of the location of the desired pass. a photo that has been ordered, etc. can be taken....